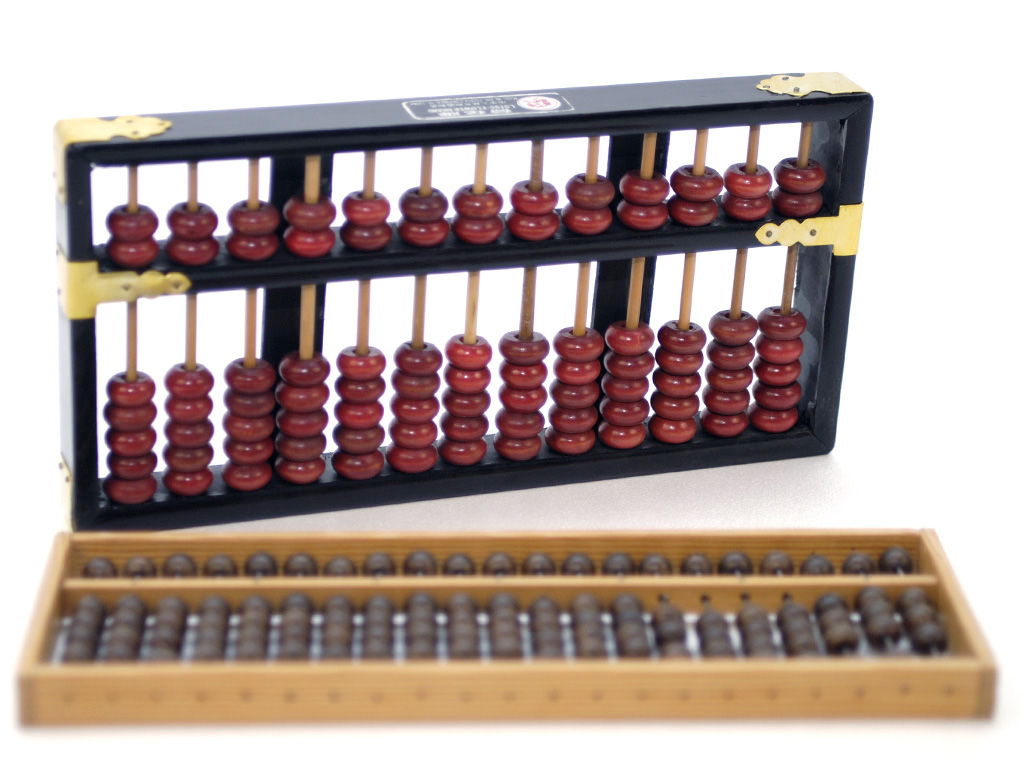

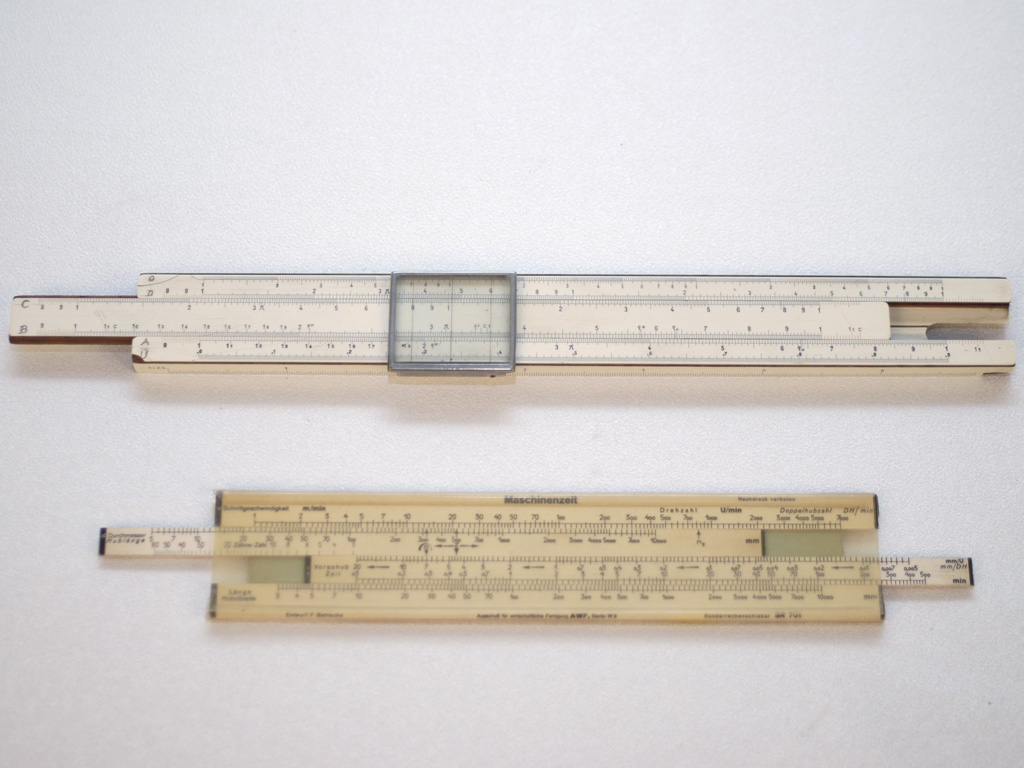










A slide rule is a analog calculation aid, for the four basic calculating modes. Especially the multiplication and division (besides root, square, logarithm and trigonometric functions or parametric calculations)
In the first 200 years after its invention the slide-rule was highly freqeunted, Since the end of the 18th century his importance was new discoveredby James Watt.
Because of the technical development in the age of the industrial revolution the slide rule became a often used instrument for technical and scientific calculations. Since that time it was a symbol for ingeneers, mathematic and physical scientists. Besides the slide rules, which are used in the school lessons or in simple applications in the revery day life, some special sliding rules were produced for special category groups like aviation, electrical and systems engineering, chemistry, millitary or for trading purposes.
Even 1624, ten years after the discovery of mathematical logarithms, the english theologian and mathematician Edmund Gunter published his thoughts about logarithmical numbers. He developedd the „Gunterscale“, which is mainly a staff with a logarithmical scale. On the begining itwas possible to realize a simple subtraction and addition.
Groundbreaking was the brainchild of Englishman William Oughtred in 1622, instead of the dividers two congruent logarithmic scale to even or circular. So the true inventor is William Oughtred. Seth Partridge also contributed to the development of the slide rule in 1654.
In 1722, Warner used first square and cube scales. Which were invented by Isaac Newton and implemented in 1775 by John Robertson. This highly practical development increases the accuracy of the reading.
The double logarithmic Exponentialskalen were invented in 1815 by the English physician and lexicographer Peter Mark Roget. They are used to simplify any kind of expotential problems. Eine große Bedeutung in der Geschichte des Rechenschiebers kommt dem Franzosen Victor Amédée Mannheim zu, der im Jahre 1850 einen einheitlichen Aufbau für den bis zuletzt verwendeten Schulrechenstab durchsetzte. Significant for the history of the slide rule is also Ameédée Mannheim, who developed and carried the actual design of the todays slide rule.
Around 1975 even schools began to use the electronical calculator instead of the slide rule, which causes the end of the using of the silde rule.
The most coveted slide rules, are the four original ones of William Oughttred, each is worth about 250000 US Dollar. They are all exposed in museum.
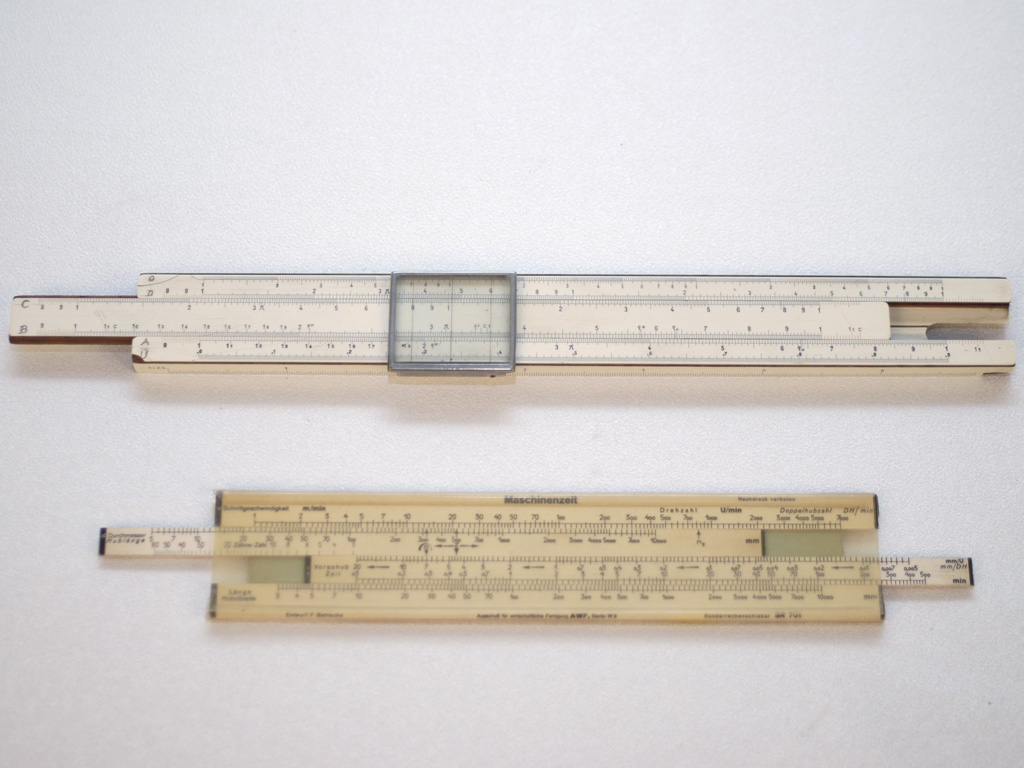
There are noumerous different slide rules. On each existing a few (mostly logarithmic) scales, having several functions. The most slide rules come with a table of the used scales. The used capital letters in the table are the common notation of the slide rules.
The scales B and C are on the slide, so they can moved in the relation of the rule. Futher there is a runs with a line to balance the different scales.
| Description | Division | Area | Comment |
|---|---|---|---|
| L | linear | [0].0 ... [1].0 | Scale of the mantissa Shows the mantissa of the decadic logarithmic of x. Works together with L and C. The nummerous has to be calculated. |
| D | logarithmic | 1 ... 10 | Main scale |
| C | logarithmic | 1 ... 10 | Main scale on the tounge |
| C | logarithmic | 10 ... 1 | Inverse of the main scale; is locatet at the tounge |
| B | logarithmic | 1 ... 100 | Second main scale on the tounge |
| A | logarithmic | 1 ... 100 | Second main scale |
| K | logarithmic | 1 ... 1000 | Scale for calculation of the volumina |
| S | logarithmic | 5.5° ... 90° | Scale for calculating the sinus of an angle. At the 'Rietz'-type often on the backside of the tounge |
| ST | logarithmic | 0.55° ... 5.5° | Scale for calculating the sinus, tangens und radian of small angles. At the 'Rietz'-type often on the backside of the tounge |
| T oder T1 (T2)) | logarithmic | 5.5° ... 45° (45° ... 84.4°) | Skala zum Bestimmen des Tangens und eines Winkels. Beim Typ Rietz oftmals auf der Rückseite der Zunge. Manchmal gibt es eine zweite Skala T2 für Winkel zwischen 45° und 84.5° |
On the example of the multiplication: It is possible to spilt the feactors of the logarithm into two logaritms and to add them. Therefore the scales D andC are logarithmic